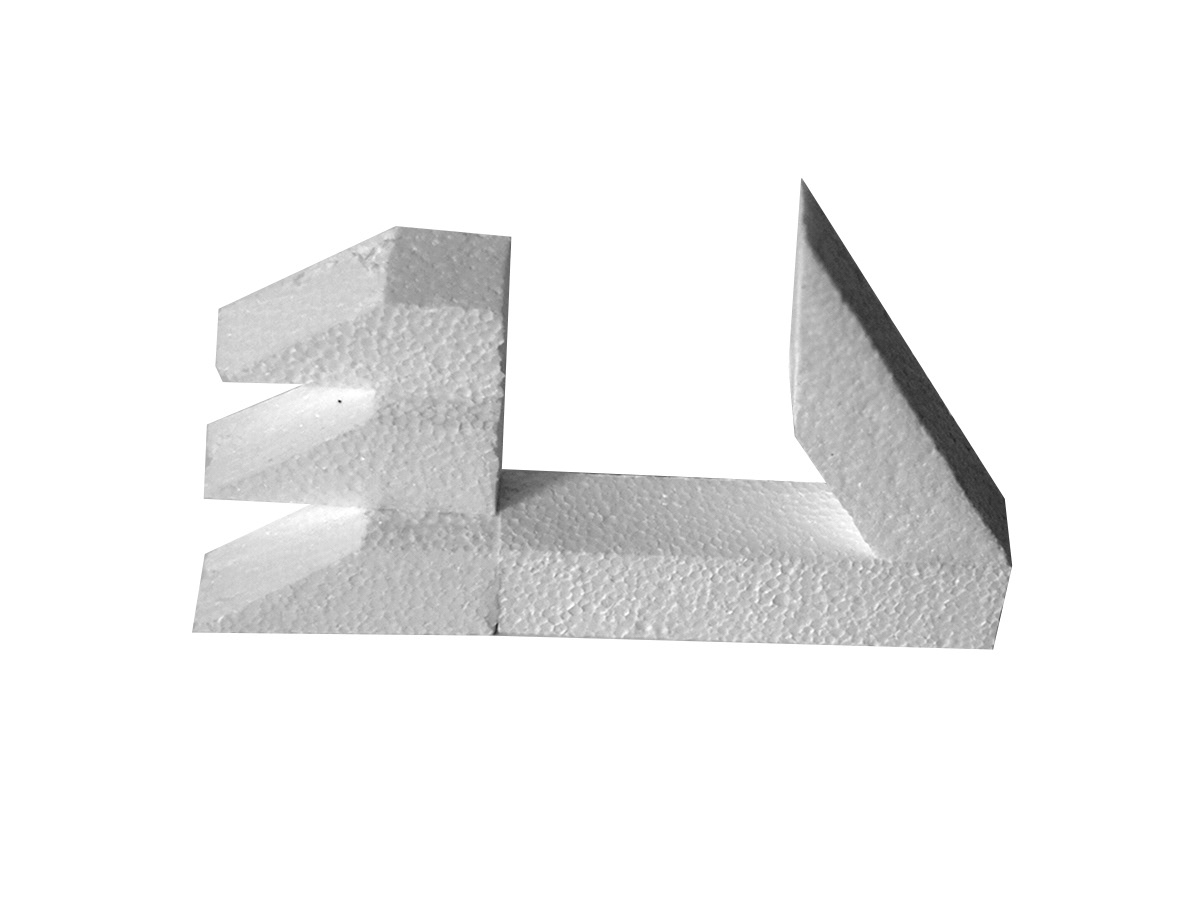
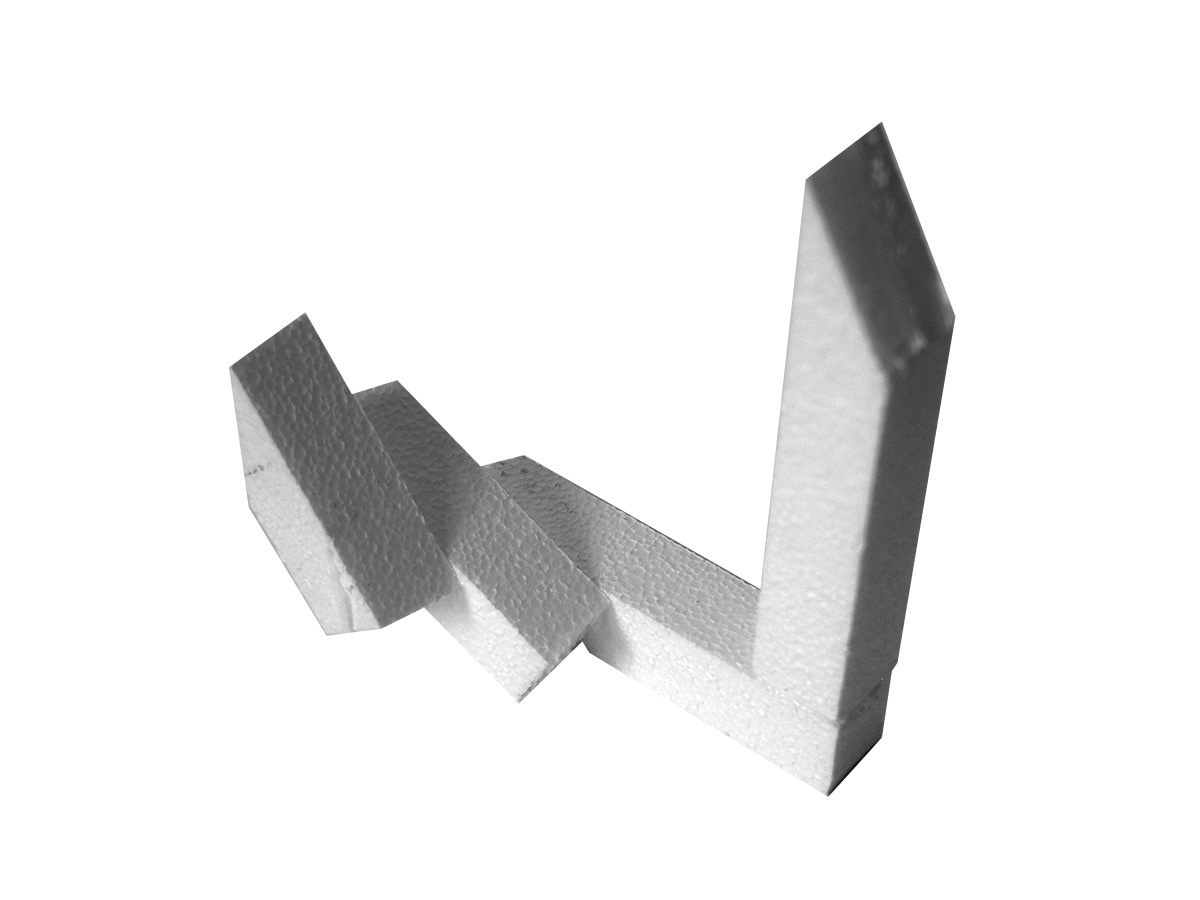
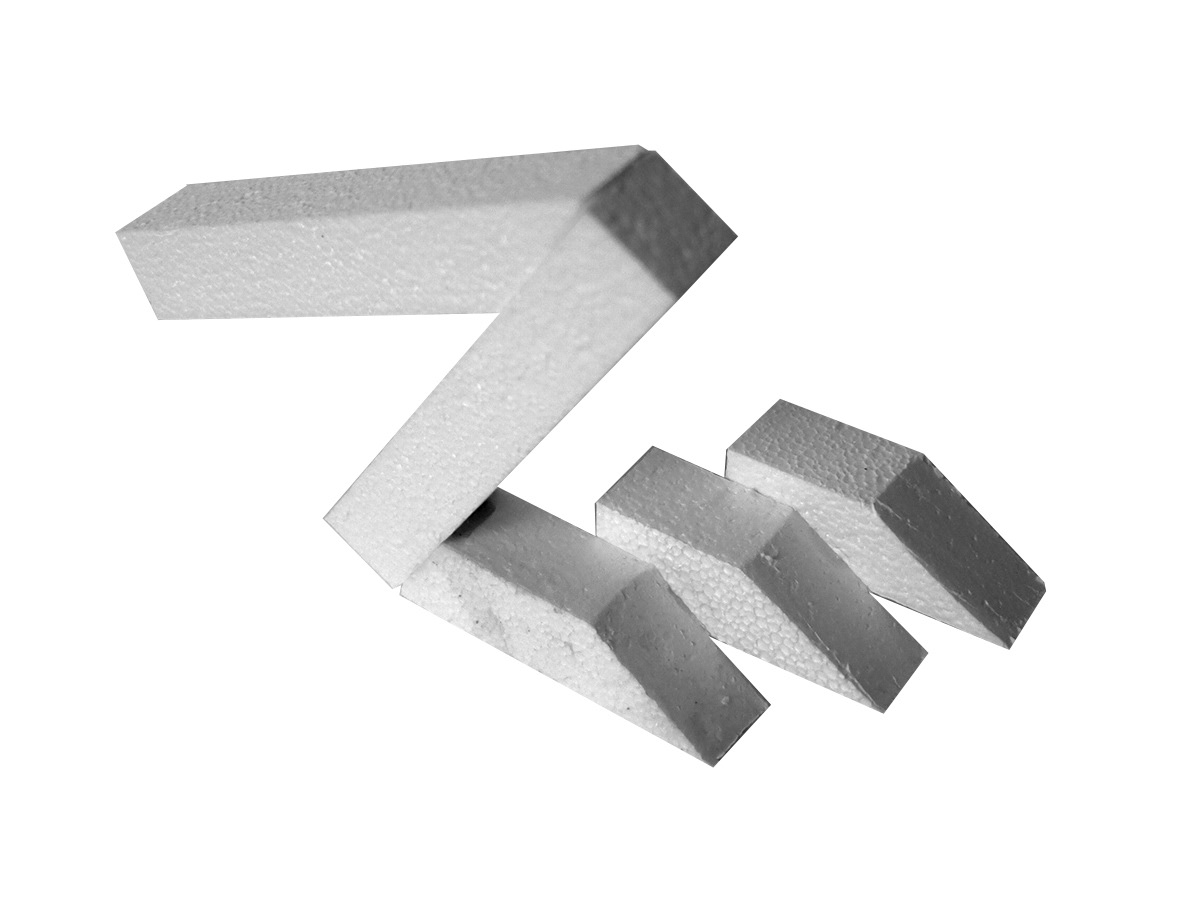
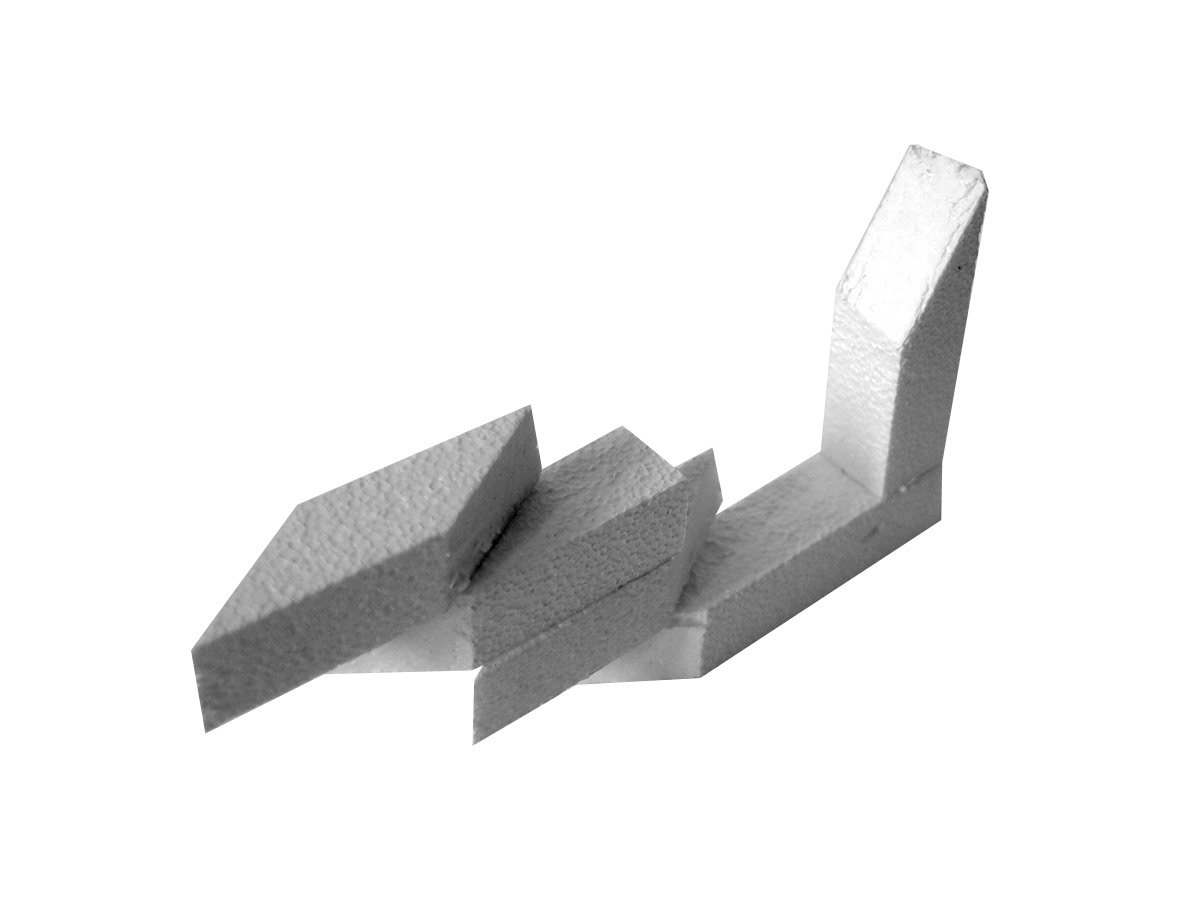
LINEAR SEQUENCE
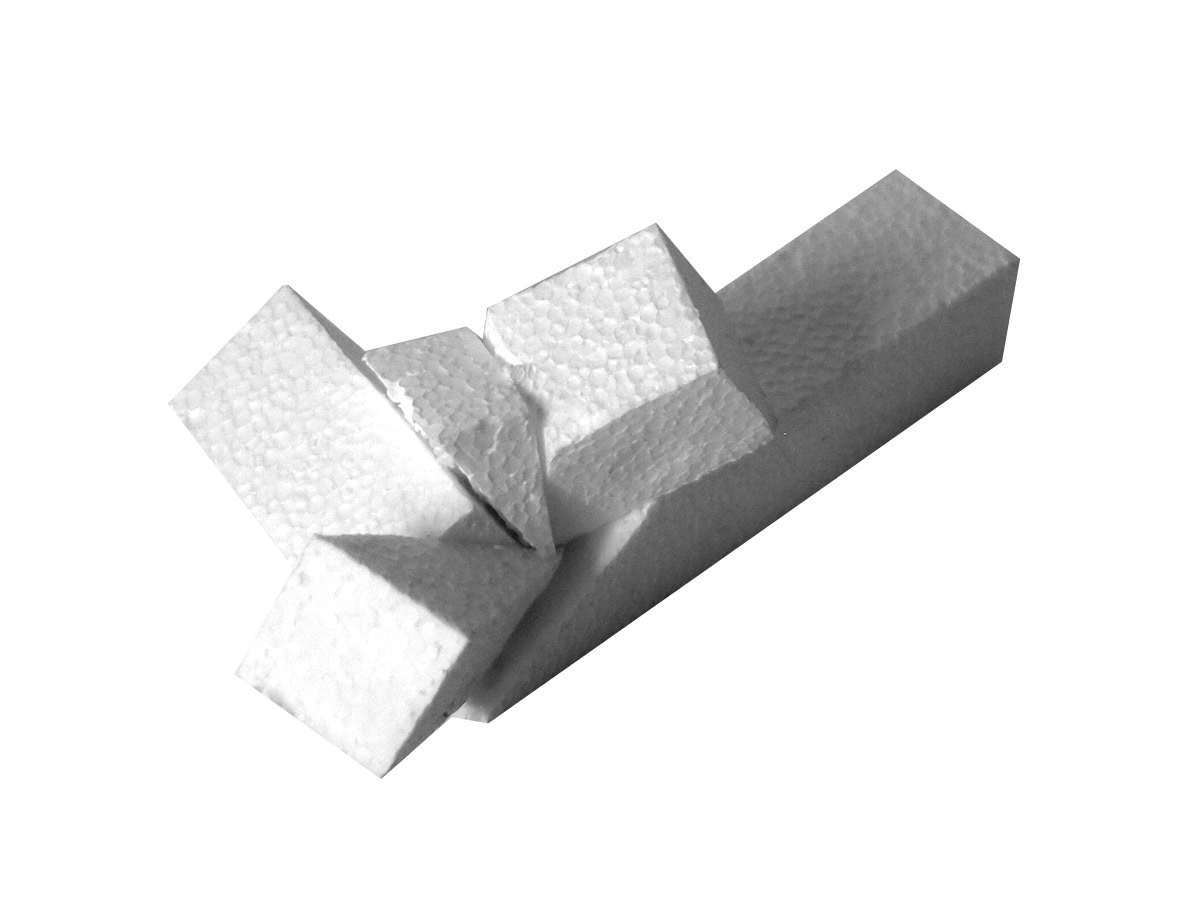
A linear sequence is defined as a set of units throughout which there is one clear direction of procession from one unit to the next. These models each contain two stages with no pre-established chronology: a stage of large-scale units in two dimensions and a stage of small-scale, identical or near-identical, stacked units which show directionality in a third dimension. Always showcasing the oblique faces of the small, stacked units, each model utilizes a differing mode of stacking, which reveals an exploration of translation, rotation, pattern, and parallelism. Some models demonstrate stacking along the ground plane, others parallel to it, and others yet orthogonal. A stack must include three oblique faces, although someimes this includes a face on a large-scale, base unit which is included in both the small-scale stack and the large-scale stage.
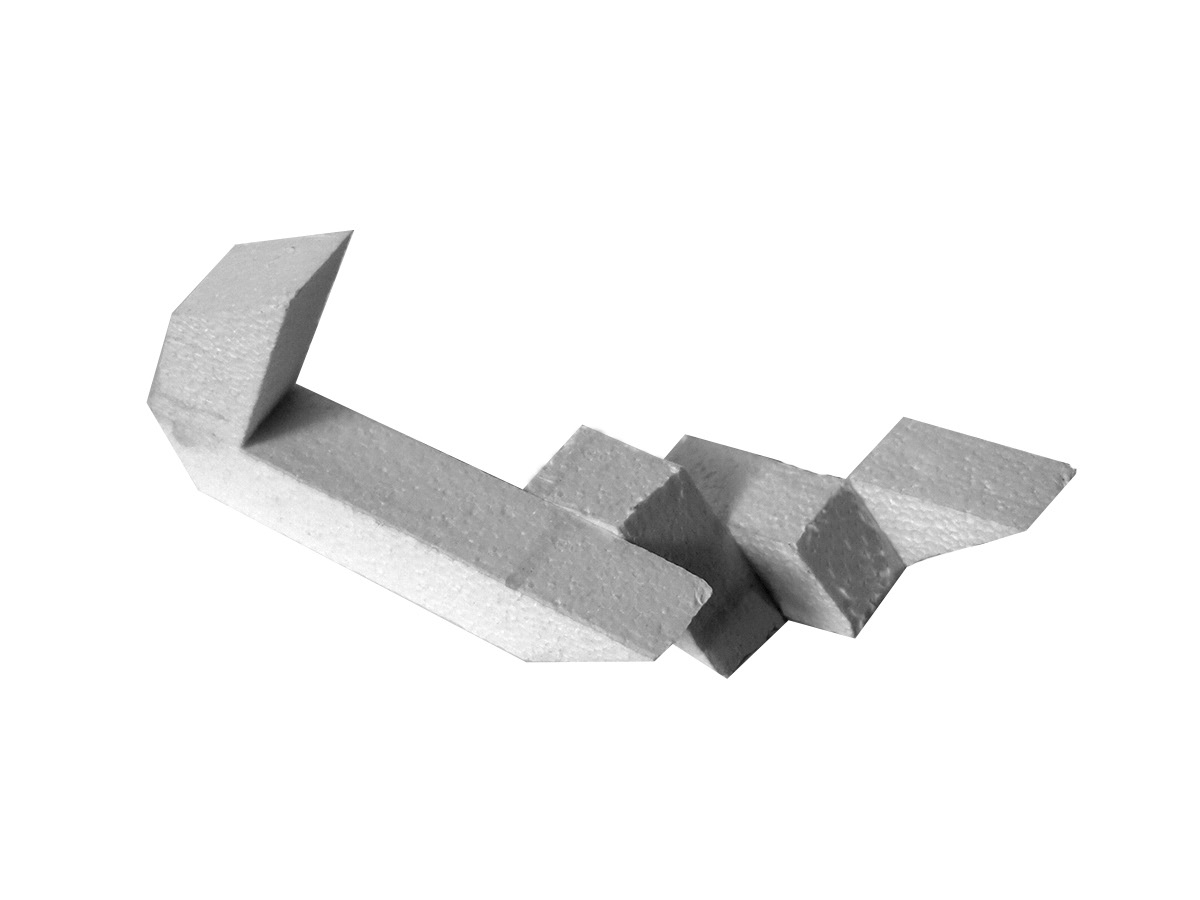




TWO-DIRECTIONAL MATRIX
A two-directional matrix exists along only one plane, utilizing directionality in the “x” and “y” dimensions but never emerging in the “z” dimension. Furthermore, it is distinguished from a linear sequence by its lack of a clear progressive direction from one unit to the next: a two-directional matrix can diverge or otherwise have more than two branches emerging from a single point in its structure. These models each utilize a set of two primary units—a long, asymmetrical trapezoid and a short rectangle—as a base to which various secondary units are attached in different ways, some symmetrical and others not. Secondary units are never attached to the oblique faces of the primary units; they are always attached to the rectangular sides. Sometimes, the base units are central to the matrix; other times, they are off to one side.






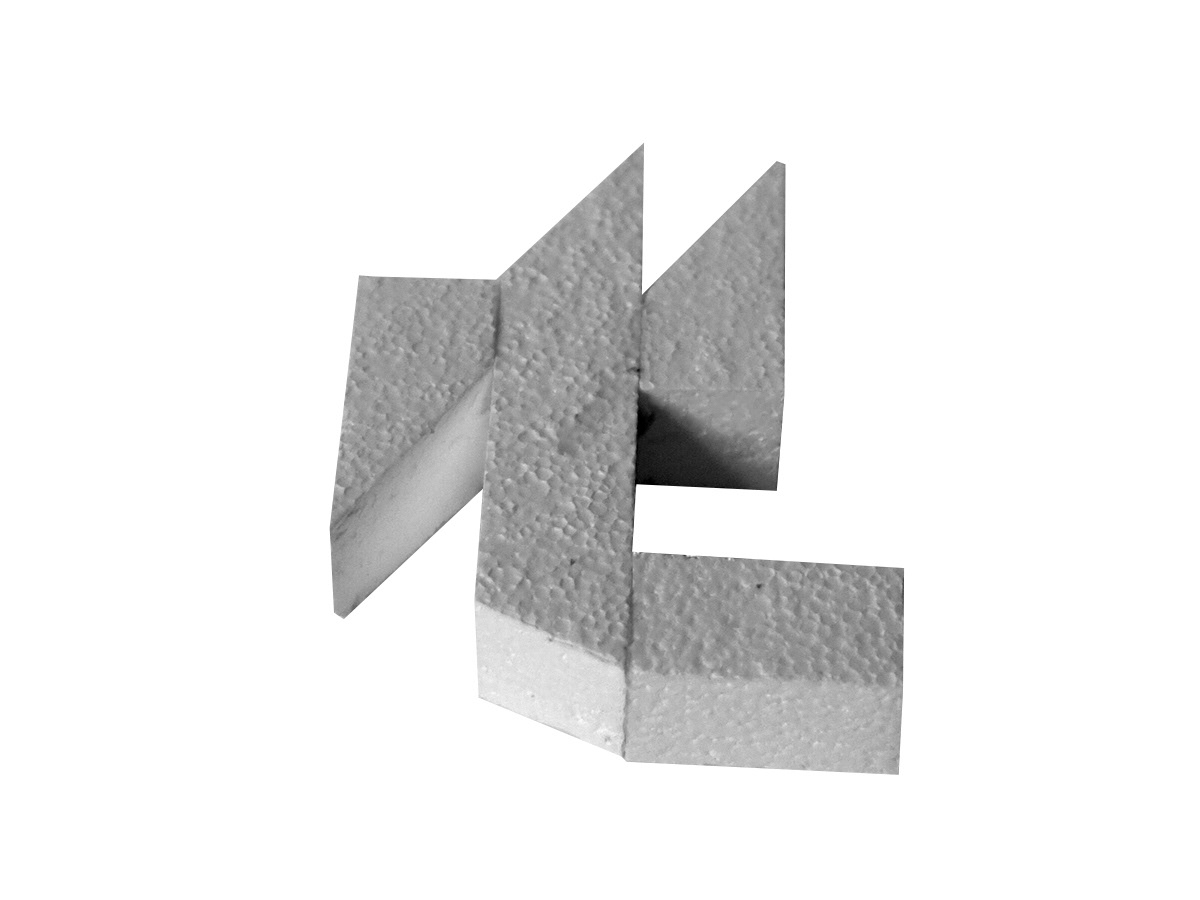


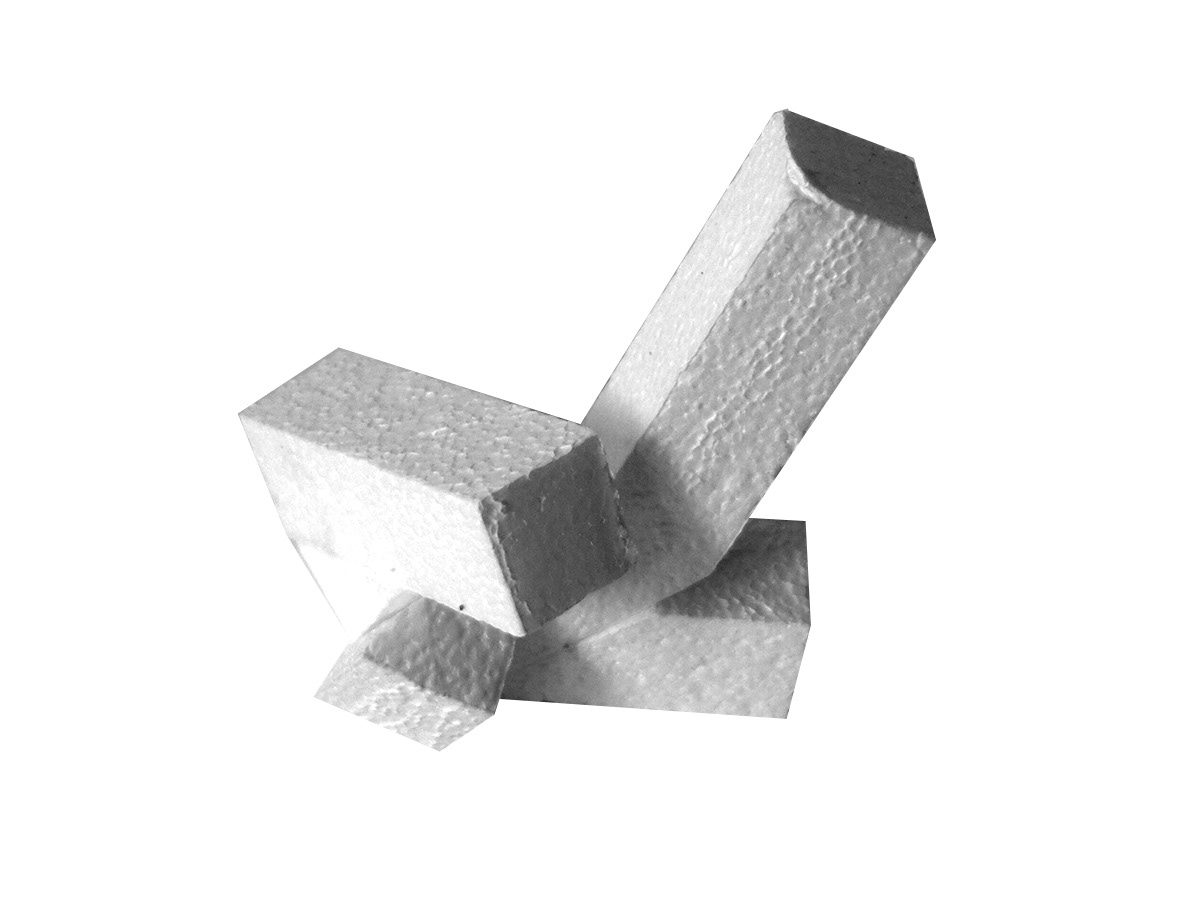
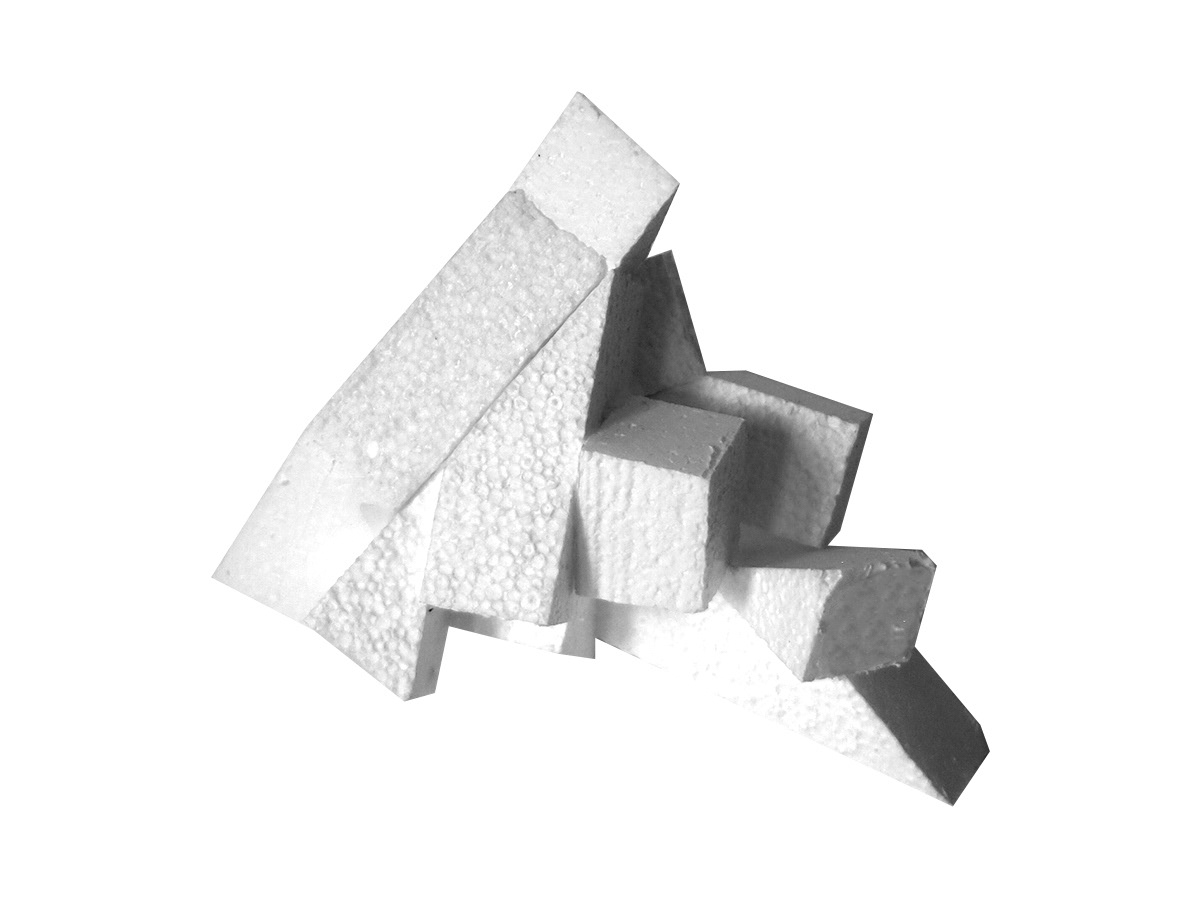
VOLUMETRIC CLUSTER
A volumetric cluster exhibits directionality in three dimensions and is distinguished by its density; its mass is all or mostly concentrated at one point in space. In these models, all units used emerge from this point. The units are arranged in such a way that showcases disorder and irrationality, imitating the formation of crystals in the natural world. The models differ in scale and number of units used; these variations alter the emphasis in the forms of these models. Models with fewer units bring emphasis to individual units and how they are attached to each other; models with more units bring emphasis to the entire form and often appear to have no clear point or method of attachment. All of the models have a base unit which exists parallel to the ground plane and which also participates in the aggregation of all the units to form the cluster.